How Teaching With Symmetry Improves Math Understanding

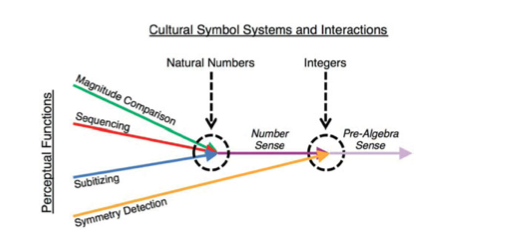
There's a part of the brain that enables us to perceive magnitude -- we can compare loudness when hearing different tones or compare the number of dots in a group at a glance. Neuroscientists have identified this region responsible for perceptual comparison (the intraparietal sulcus) as linked to symbolic comparisons, including integers in math. That discovery led scientists to realize that symmetry plays a big role in how humans compare integers.

Building on this background research, Stanford education researchers tested a teaching strategy explicitly focused on using symmetry to teach integers to fourth-graders. They wanted to see if recruiting the visual symmetry parts of the brain would improve students’ facility and understanding of the concept. Their findings, published in "Cognition and Instruction" in May, indicate that teaching with symmetry could have a big impact not just on students’ understanding of integers, but also on more advanced concepts that go well beyond the scope of instruction as well.

“Finding a fundamental discovery in neuroscience about how the brain is processing these numbers led us to realize the instruction needs to change,” said Daniel Schwartz, director of Stanford’s AAALab and one of the authors of the study.

When people are asked to find the distance between a negative integer and a positive integer oriented in a blank space, they are able to do so much more quickly when the numbers are spaced around zero. For example, people can more quickly calculate the difference between -2 and 3 than they can -5 and 3. Furthermore, an fMRI scan of the brain while this calculation is taking place reveals that visual symmetry is in play.

In math instruction “nobody had been focusing on the significance of zero, which is the reflection point,” Schwartz said. There are two common instructional methods used to teach integers in current curricula: the first uses distances on a number line, asking students to jump forward and backward between integers. The other uses the idea of cancellation, where students are taught that positive and negative numbers represent opposite quantities that cancel each other out.

The AAALab researchers split students into three groups and used these two accepted instructional models to teach integers to two of the groups. The third group used a custom-built manipulative that bends at zero so students could visually see the symmetry. All the groups played with hands-on manipulatives for a while to get the hang of the idea and then practiced problems using the condition they had been taught on computer games to increase their facility. Schwartz called this practice time “increasing the dosage.” At the end, researchers gave them all the same test.

Students in all three conditions performed well on the straightforward symbolic problems on the test, the core of the material that had been taught. Schwartz said that’s good because it shows that all the instruction given was good, and variables like poor teaching did not influence the results.

“The difference showed up on bigger problems, harder problems, where you really had to start organizing the numbers in your head,” Schwartz said. “You couldn’t just do fact retrieval.”

For example, one question asked: In a Tug of War game, the red team has five people and the blue team has three people. The blue team thought that was unfair, so they added four more players to their team. How many players does the red team now need to make the teams even? Another question asked about negative and positive fractions. Even without understanding fractions, students correctly found the mirrored version.

“On these problems where you have to learn new things, suddenly you see that symmetry stuff showing up and it’s basically because kids had a much better structure to organize new ideas and new information,” Schwartz explained.

And, even better in his mind, the test included a few questions that would be hard to solve using the symmetry method the test group learned. As Schwartz hoped, the students who were using the symmetry methods struggled with those problems. He said that’s good because it proves that the intervention improved their knowledge in a specific way, not just generally. The prowess the symmetry condition students showed on more difficult tasks and even pre-algebraic tasks was not a halo effect.

“It really showed [the intervention] improved them on many things we really care about and made them worse at something that we don’t care about,” Schwartz said.

The study points to a clear instructional method that could improve student learning not just on simple integer problems, but on many other concepts using symmetry. Students often struggle with the idea of equivalence, but recruiting symmetry could provide the structure they need to grasp this concept that is so central to algebra.

The next step will be for someone to go out and build manipulatives that bend at 0, like the ones Schwartz’s lab fashioned for this experiment. He thinks it’s possible a computer program could simulate this idea as well, but it would need to make sure the student’s eyes were following the hands and focusing on the point of inflection.